2x−y=−5
y=1−3x
1,-2
2y < 4x - 6 and y < 1/2x + 1
3,3
X=Y+5
3x+2y=5
(3,-2)
x-3y=12
y=1/4x-7
Consistent and Independent
What vocabulary term means a system of equations with at least one ordered pair that satisfies both equations.
Consistent
3x−y=13
3y−2x=−4
5,2
y > 2/3x - 7 and x < -3
-3,-9
x=-2y+5
3x+3y=-3
(-7,6)
8x+2y=18
y=9-4x
Consistent and dependent
What vocabulary term means a consistent system of equations with exactly one solution.
Independent
5u−6v=−2
7u+18v=2
-1,1/2
3x - 2y < 2 and y > -1
0,-1
x+y=9
x-y=5
(7,2)
x-5y=10
2x-10y=30
Inconsistent
What vocabulary term means a consistent system of equations with an infinite number of solutions.
Dependent
8x−5y+16=0
1x+3y−17=0
1/2,4
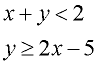
2.5,-.5
2x=-3y+16
5x-4y=-6
(2,4)
Something with one solution and and the slopes are different means it is Consistent and Independent True or False.
True
What vocabulary term means a method that involves eliminating a variable by combining the individual equations within a system of equations.
Elimination
4(x+2)−7(x−y)=7
7(x+y)+10(x−2)=79
5,2
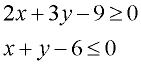
9,-3
3x+y=10
-4x-2y=2
(11,-23)
If there are no solutions and the slopes are the same then it is Consistent and Dependent True or False
False
What vocabulary term means a process of solving a system of equations in which one equation is solved for one variable in terms of the other.
Substitution