Graphing
Substitution
Elimination
Interpret
Miscellaneous
100
Find the solution(s). 
(4,5)
100
Solve.
x = 2
y = 4x -7
(2,1)
100
Solve.
5x + 5y = 5
-8x - 5y = -11
(2, -1)
100
One line shows combinations of dimes and quarters that are worth $3 altogether. The other line shows combinations of dimes and quarters that total to 12 coins.
How many quarters and dimes would you need to have both 12 coins and $3 at the same time?
12 quarters and 0 dimes
100
Which method should you use to solve this system? What is the first step?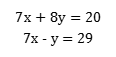
Use elimination and multiply one of the equations by -1
200
Find the solution(s).
(1, -2)
200
Solve.
y = 8
y = x + 1
(7,8)
200
Solve.
-6x + 2y = 12
-2x -2y = 20
(-4, -6)
200
Traditional incandescent light bulbs are cheaper to purchase, but they use more energy compared to LED bulbs.
Traditional incandescent bulbs cost about $1 each, and 12 cents per week to use. LED bulbs cost about $5 each, and 2 cents per week to use.
Which light bulb costs $10 in 75 weeks?
Incandescent
200
If two lines are perpendicular, how many solutions will there be?
One
300
Find the solution(s).
(0,0) and (1,1)
300
Solve.
y = -2x + 4
y = 6x - 20
(3, -2)
300
Solve.
-2x + 9y = -4
2x + 3y = -20
(-7, -2)
300
What is the initial cost of Des-Mobile?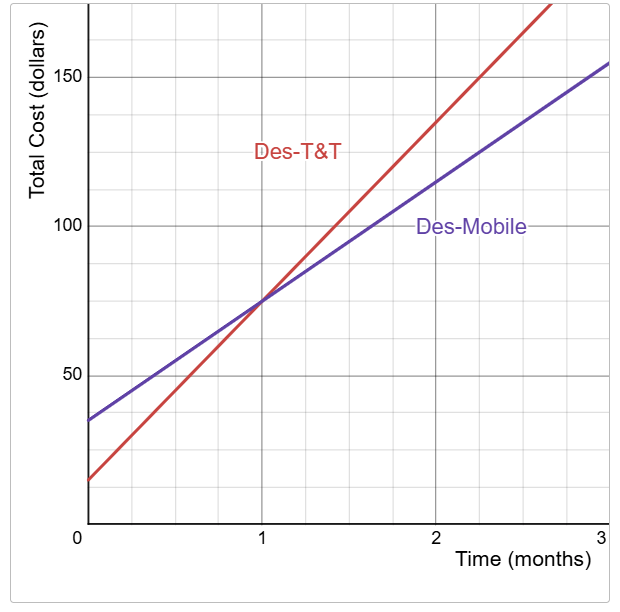
Around $40
300
How many solutions are there if you get something like this?
14 = -3
Zero
400
Graph the following equations on your white board. Find the solution(s).
y = -2x + 1
y = x - 2
(1,-1)
400
Solve.
4x - 4y = 0
y = 2x + 6
(-6,-6)
400
Solve.
7x + 8y = 20
7x - y = 29
(4, -1)
400
Who is at 20 meters after 5 seconds?
Neither of them
400
How many solutions are there if you have two coinciding lines?
Infinite
500
Graph the following equations on your white board. Find the solution(s).
y = 8
y = x+1
(7,8)
500
Solve.
x = 5
x = -2
No solution (the lines are parallel).
500
Solve.
4x + 2y = 10
-2x + y = 9
(-1, 7)
500
Who can make 50 signs faster? How long did it take them?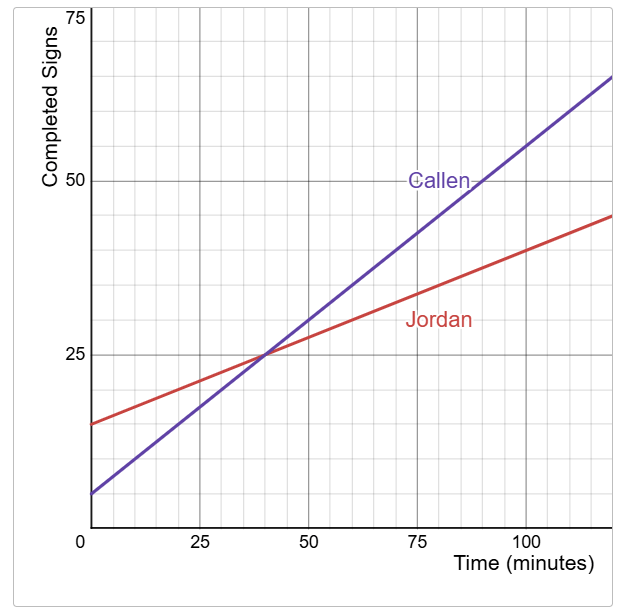
Callen made 50 signs in about 90 minutes
500
How many solutions are there to a system with three parallel lines?
Zero