Reflections
Translations
Rotation
Dilation
All
100
What is the reflection , across the x axis, of the triangle with the points A(5,10) B(-2,4) C(5,0)?
A(5,0) B(5,-10) C(-2,-4)
100
 Translate the point 2 units up and 2 units to the right.
(3,1)
100
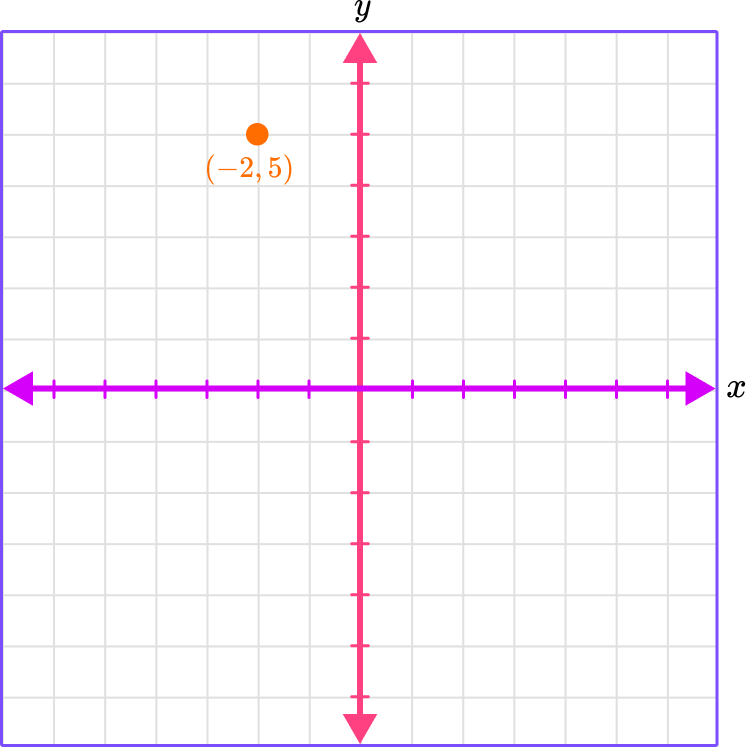Rotate the point 90 degrees clockwise.
(5,2)
100
 Dilate this figure by 2.
A'(0,8) B'(6,4) C'(6,-2) D'(-6,-2) E'(-6,4)
100
The point on this graph is (2,3). Reflecting this point over the X axis, what is the new point?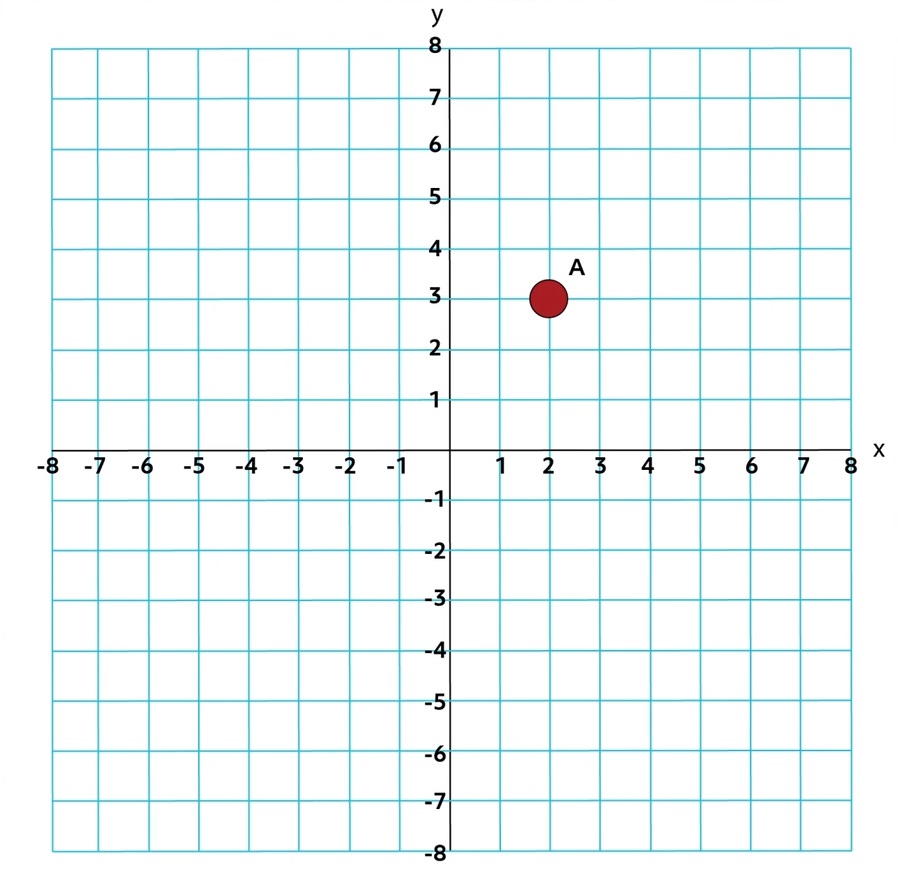 
(2,-3)
200
 Reflect this image across the X-axis
(-3,-2) (5,-3) (3,-6)
200
Translate triangle DEF 1 unit right, and 3 units down. what are the new points? D (-5,1) E (-2,5) F (1,-1)
D' (-4,-2) E' (-1,2) F' (2,-4)
200
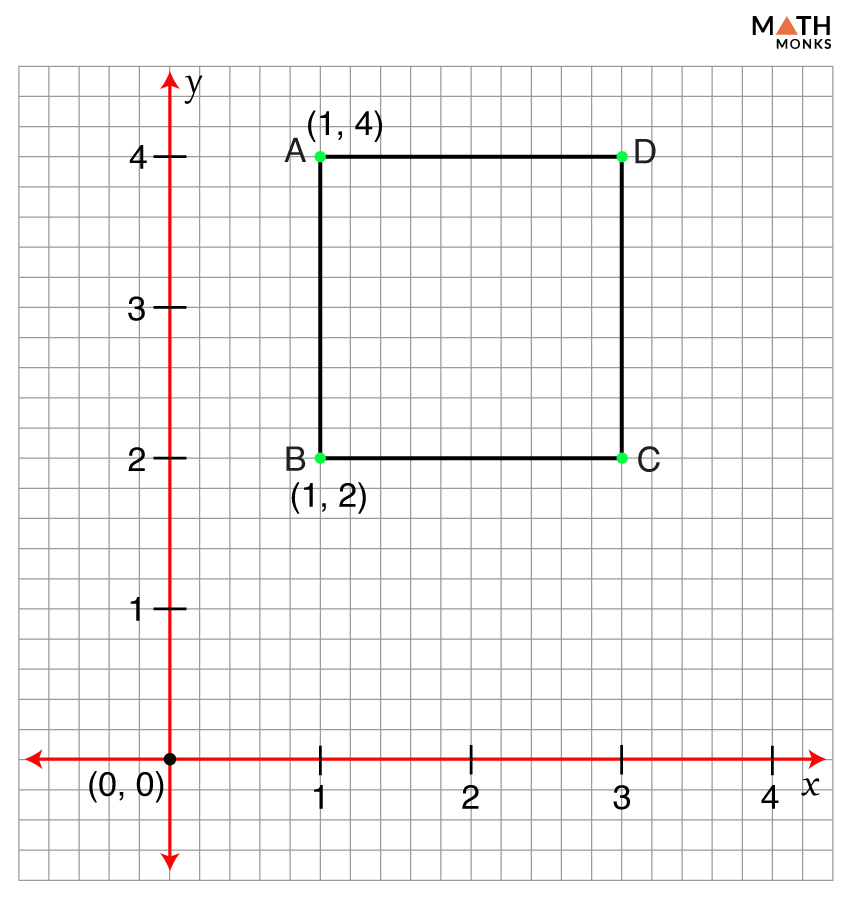Rotate the square 180 degrees counter clockwise.
A' (-1,-4) B' (-1,-2) C' (-3,-2) D' (-3,-4)
200
A'(4,20) B'(16,-8) C'(-20,4)
200
Translate triangle ABC up 3 units and 8 units to the left. A(1,3) B(5,2) and C(3,2)
A"(-7,6) B"(-3,5) C"(-5,5)
300
A trapezoid labeled A (2,8) B (6,8) C (8,3) D(1,3) is reflected across the y-axis. What are the new coordinates?
A' (-2,8) B' (-6,8) C' (-8,3) D' (-1,3)
300
Translate up 3 units, left 4 units.
A' (1,6) B'(-6, 6) C' (-6,-1) D' (1,-1)
300
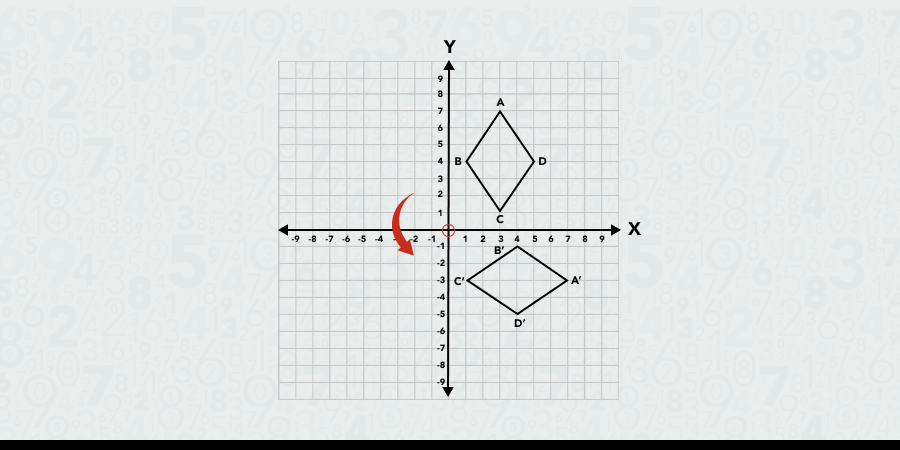Describe what rotation this is.
270 degrees clockwise rotation.
300
 Write the rule for this dilation.
The figure has a scale factor of 4.
300
Rotate this point 180 degrees counterclockwise
(-1,-1)
400
The vertices of ABC are A (-5,5) B (-2,5) C (-2,3). If ABC is reflected across the line y= -1, find the coordinates of the vertex C'.
C' (-2,-5)
400
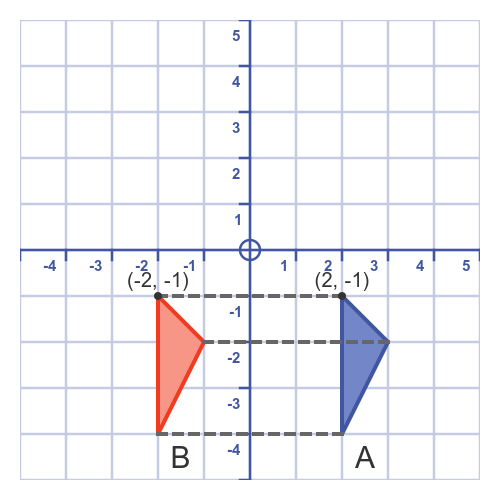Describe the translation of the image.
4 units to the right.
400
JAR has vertices of J(4,5) A(6,4) and R(5,2). What are the new points after rotating it 180 degrees clockwise?
J'(-5,-4) A'(-6,-4) R'(-5,-2)
400
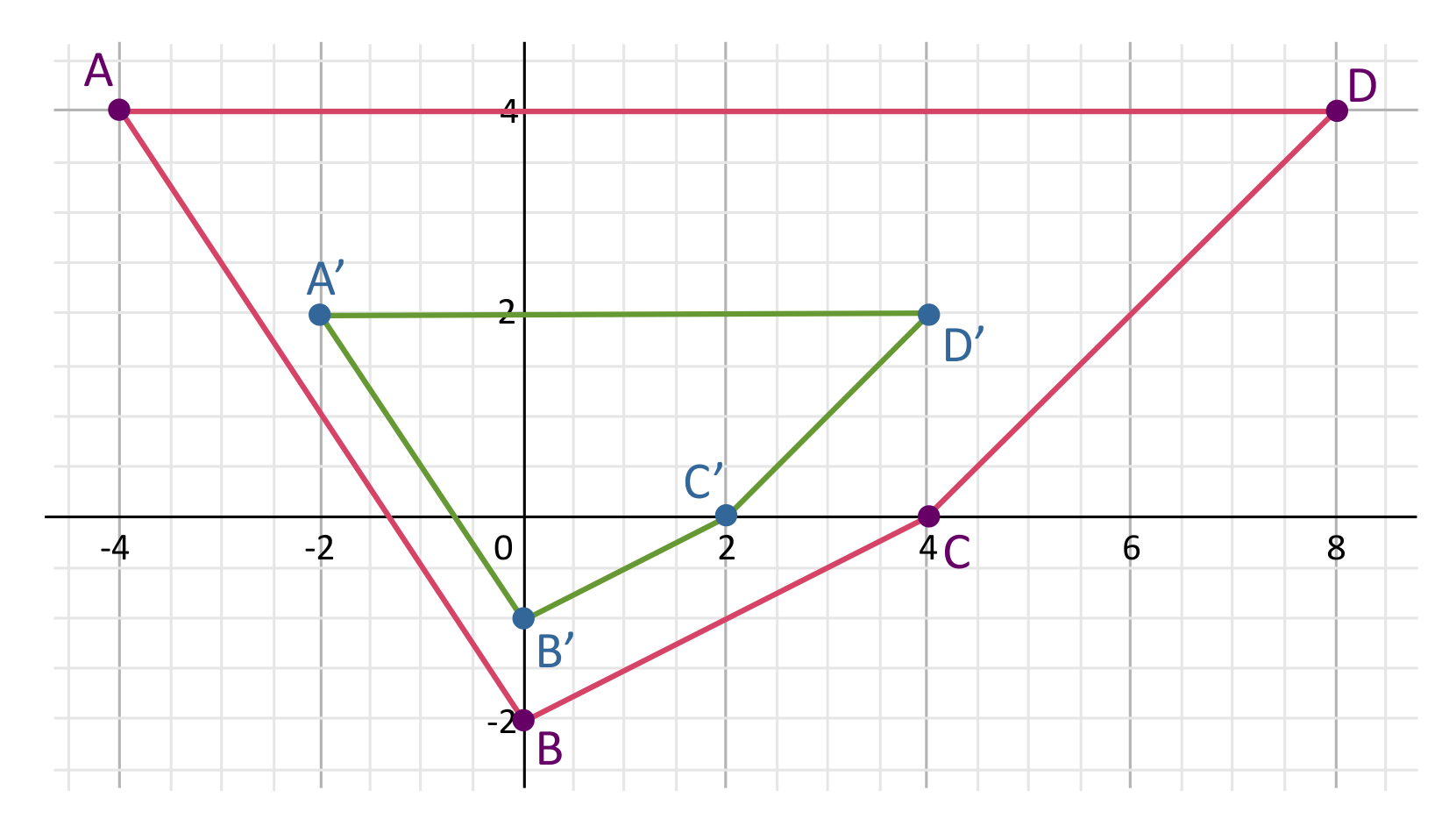Explain the dilation of this figure.
This figure has a scale factor of 1/2.
400
Reflect this point over the Y-axis. Then, rotate the new points 90 degrees clockwise.
(2,1)
500
DOUBLE JEOPARDY!!!!
The vertices of ABC are A (-5,5) B (-2,4) C (-4,2). ABC is reflected across the y-axis and then reflected again across the x-axis to produce the image A'B'C'. What are the coordinates of A'B'C'?
A' (5,-5), B' (2,-4) and C' (4,-2)
500
DOUBLE JEOPARDY!!!!!
The vertices of pentagon VWXYZ are V(4,5) W(6,5) X(6,7) Y(5,8) and Z(4,7).
a. What are the points after a translation of 10 units left and 2 units down?
b. Estimate the distance between V and V' to the nearest tenth?
a. V'(-6,3) W'(-4,3) X' (-4,5) Y' (-5,6) Z' (-6,5)
b. About 10.2 units.
500
Rotate the points ABC A(5,3) B(5,7) C(7,2) 90 degrees clockwise. Then rotate those new points 270 degrees counter clockwise.
A''(-5,-3) B''(-5,-7) C''(-7,-2)
500
Dilate the points A(7,2) B(4,3) C(6,2) by 4, then dilate new points by 1/2. What are the final points of the figure?
A''(14,4) B''(8,6) C''(12,4)
500
Dilate the points A(5,2) B(3,3) C(3,2) by 2. Then, rotate the new point 180 degrees clockwise. What are the new points?
A"(-10,-4) B"(-6,-6) C"(-6,-4)