General Sequences and Series
Arithmetic Sequences
Geometric Sequences
Arithmetic Series
Geometric Series
100
What is the difference between a sequence and a series?
A sequence is an ordered list of numbers, whereas a series is the sum of a given number of terms in a sequence.
100
Write the formula for the given arithmetic sequence:
-2, 2, 6, 10, 14, ...
an = -2 + (n-1) 4
or
an = 4n - 6
100
For the given sequence, find the common ratio
1/2, 2, 8, 32, ...
r = 4
100
Find the summation of this series:
an = 2n + 1 for the first 5 terms.
35
100
Is the following a geometric series?
3 + 12 + 21 + 30, ...
No, this is arithmetic
200
What is the difference between an arithmetic and a geometric sequence?
An arithmetic sequence is a sequence that has a common difference (the same number gets added each time).
A geometric sequence is a sequence that has a common ratio (the same number gets multiplied each time).
200
Given that a1 = 7 and the common difference is -2, find a6
a6 = -3
200
Write the formula for the given geometric sequence:
32, 16, 8, 4, ...
an = 32 (1/2) n-1
200
Determine whether the following series converges or diverges and provide a reason why.
18.75+17.50+16.25+15.00+....
Diverges because it is an infinite arithmetic series.
200
Determine whether the following series converges or diverges and provide a reason why.
65+13+2.6+0.52+...
Converges because infinite geometric with r=0.2 and therefore has a sum.
300
Find the sum of the following series:
2, 11, 20, 29, 38
100
300
For the given sequence, find the common difference and the 10th term.
9, 12, 15, 18, ...
d = 3
a10 = 36
300
Given that a1 = 3 and r = 2, find a6.
a6 = 96
300
Determine the number of terms in the series:
-1 + 2 + 5 + ... + 68
n = 24
300
Find the sum of the geometric series:
486 + 162 + 54 + ... + 2
728
400
What is the next number in the pattern?
1, 1, 2, 6, 24, ?
120
400
Find the 12th term of a sequence that has a first term of 5 and a common difference of 7
a12 = 82
400
Given the formula for the geometric sequence, list out the first 5 terms:
an = (-2)-n
-1/2,1/4,-1/8,1/16,-1/32
400
Evaluate the following series:
a1 = 42, an = 146, n = 14
1316
400
Evaluate the given series:
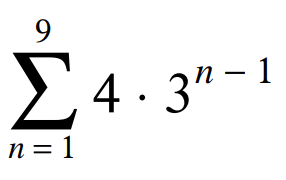
S9 = 39,364
500
Write a formula for the given sequence:
-2, 6, -18, 54, ...
an = -2(-3)n-1
500
Find the sum of the following series:
2 + 7 + 12 + 17 + ... 52
297
500
Find the sum of the following series:
10+5+5/2+...
20
500
Evaluate the given series.
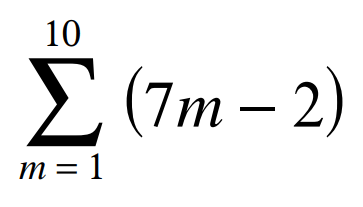
S10 = 365
500
-425/1024 or 0.415