Probability
Reading Two-Way Tables
Probability with Venn Diagrams
Probability with Two-Way Tables
Speed Round
100
An experiment involves tossing three coins and counting the number of heads. Here are the results
after running the experiment 100 times. Find the experimental probability of obtaining less than 2 heads.
51/100
100
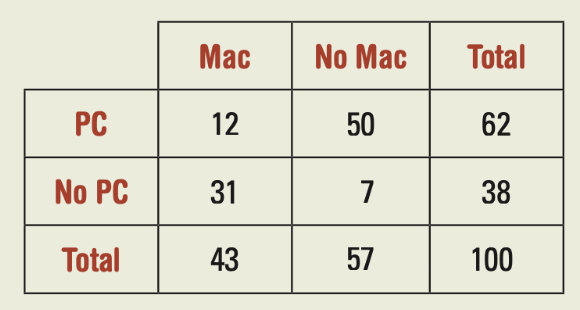
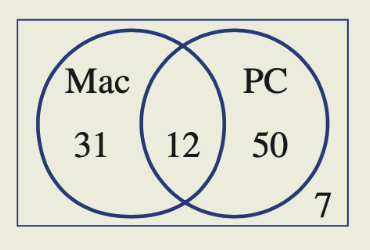
How many people like skateboards and snowmobiles?
80
100
What is the probability that a randomly chosen student likes both mathematics and physics?
40/100
4/10
2/5
100
Draw this information as a Venn Diagram:
100
What is the probability that a randomly chosen student eats breakfast and is aged 10 - 13?
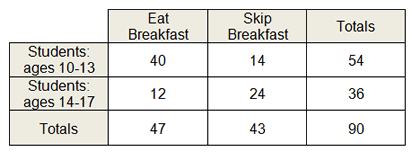
40/90
4/9
200
A bag contains 3 blue marbles and 1 yellow marble. Two marbles are drawn from the bag, without replacing the marble from the first draw.
Find the probability of selecting two blue marbles.
6/12 = 1/2
200
How many people in total were surveyed?
240
200
What is the probability that a randomly chosen person does not like cookies or ice cream?
8/80
1/10
200
What is the probability that a randomly chosen person likes snowmobiles?
105/160
21/32
200
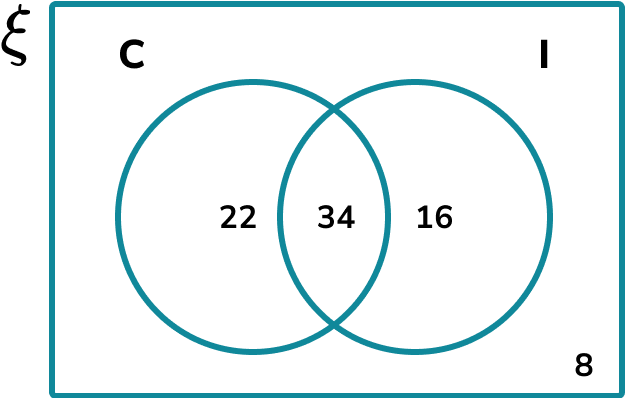
What is the probability that a randomly chosen student likes English or Mathematics or Both?
57/64
300
Find: P(B given A)
2/5
300
How many people in total like football?
42
300
Draw the information in a Venn Diagram:
"From a class of 25 students, 12 enjoy cricket (C), 14 enjoy netball (N), 6 enjoy both cricket and netball and 5 enjoy neither sport."
300
What is the probability that a randomly chosen person likes football and likes chocolate?
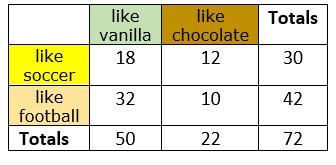
10/72
5/36
300
How many people in total like Maths?
32
400
In a chemistry class there are 5 boys and 7 girls. Two students are asked to come and help with an experiment.
If the first student was a boy, what is the probability that both students chosen were boys?
4/11
400
How many people like snowmobiles but do not like skateboards?
25
400
Given a student likes Mathematics, what is the probability that they also like physics?
40/70
4/7
400
Given a students eats breakfast, what is the probability that they are aged 14-17?
12/47
400
A letter is randomly chosen from the words MISS NGUYEN. Find the probability that an 'N' is NOT chosen.
P(not N) = 8/10 = 4/5
500
37 Scouts went hiking. 17 got lost, 19 got blisters, and 9 got both lost and blisters. Find the probability that a randomly selected Scout got blisters, given that they were not lost.
10/20 = 1/2
500
What fraction of the people who like soccer, like vanilla?
18/30
500
What is the probability that a randomly chosen student likes English, given that they also like Mathematics
19/32
500
What is the probability that a person likes chocolate, given that they like soccer?
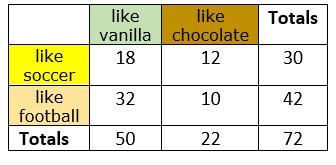
12/30
6/15
2/5
500
How many people like vanilla and like football?
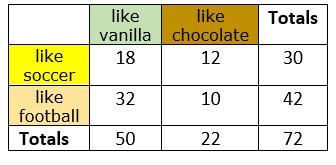
32