Area, Arc Length
Disk/Washer Method
Shell Method
Cross Sections
Mrs. Price trivia
100
Determine the area of the region bounded by
y=e^x, y=0, x=1 and x=3
e^3-e≈17.367
100
Find the volume of the solid formed when the region bounded by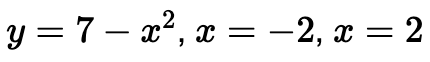 
and the x-axis is revolved about the x-axis
421.392
100
Find the volume of region V when: y=x3/2, y=0, x=1, about the y-axis
V=1.795
100
Find the volume of the solid bounded by the graph of y=x1/2 , x=4, and the x-axis using cross sections of squares with a base that is perpendicular to the x-axis.
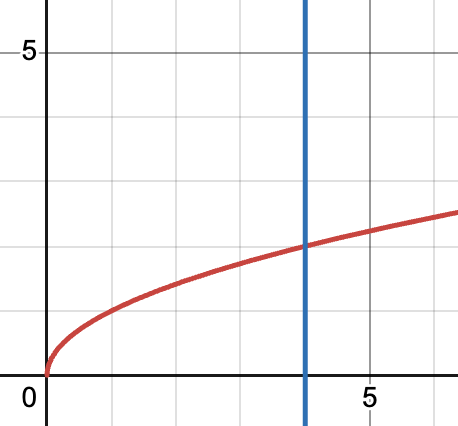
V=8
100
What are the names of Mrs. Price's two boys?
Jeffrey and Daniel
200
Determine the area bounded by the curves:
8.0435
200
Let R be the region in the first quadrant bounded by the graph of y=sqrt(x-1) , the x-axis, and the vertical line x = 10. What is the volume of the solid generated by revolving R about the y-axis?
V=723.822 or 723.823
200
Find the volume of region V when: y=
root2(x-2), x=0, y=5
Around the y-axis
V=1138.827
200
The base of a solid is the region in the first quadrant bounded by the y-axis, the graph of y=tan-1 x, the horizontal line y=3, and the vertical line x=1. For this solid, each cross-section perpendicular to the x-axis is a square. What is the volume of the solid?
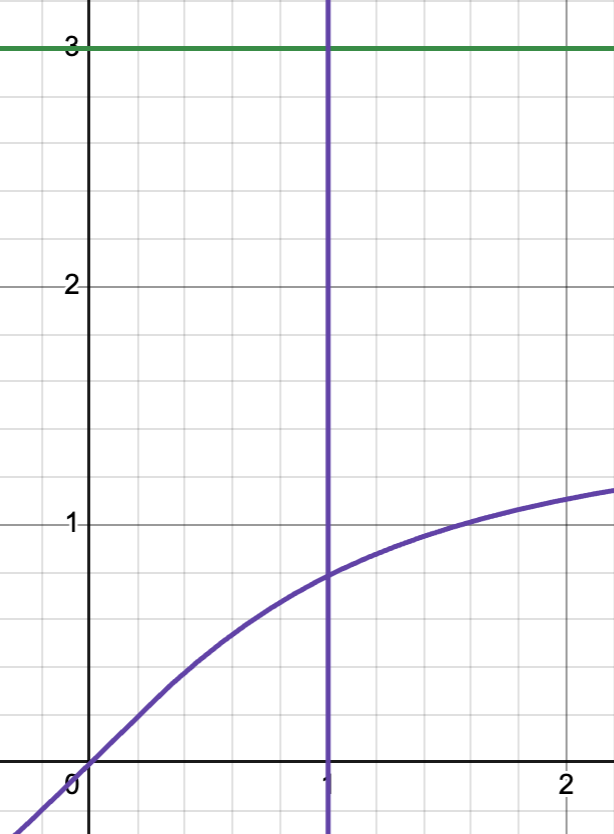
V=6.612
200
Name any university Mrs. Price has attended
Michigan State
Georgia State
UW Stout
Hamline
Minnesota State Moorhead
300
Determine the length of the arc of the top half of the curve with the equation
y^2=x^3
from x = 1 to x = 4
7.6337
300
Find the volume of the solid generated by rotating
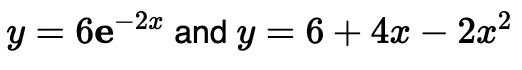
from x = 0 to x = 1 about the line y = -2
201.8647
300
Find the volume of region V when: y= 4x-x2, y=3 about x=1
V= 8.377 or 8.378
300
Find the area of the solid bounded by the x-axis, y-axis, and the line y=4-x/2 using cross sections of semicircle that are perpendicular to the x axis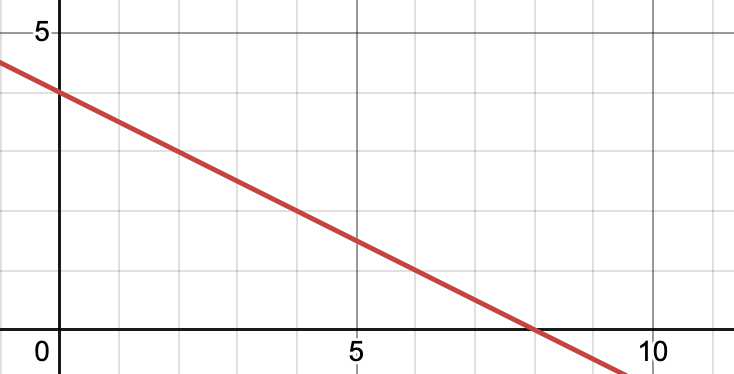
V=16.755
300
Name at least 2 of Mrs. Price's pets
Chief, Captain Awesome and Cobbler
400
Determine the area of the region bounded by
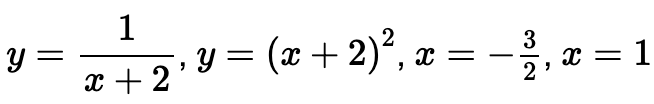
7.9695
400
Let R be the region in the first quadrant bounded by the graph of y = tan x, the x-axis, and the vertical line x = 1. What is the volume of the solid generated when region R is revolved about the vertical line x = 1?
V=1.178
400
Find the volume when: y=x2+1, x=0, y=5 about the y-axis
V=25.132 or 25.133
400
The base of a solid is the region in the first quadrant between the graph of y=2x and the x-axis for 0≤x≤1. For the solid, each cross section perpendicular to the x-axis is a quarter circle with the circle’s center on the x-axis and one radius in the xy-plane. What is the volume of the solid?
V= 1.047
or
V=pi/3
400
What was the best concert Mrs. Price saw in the past year?
I would accept either Asia or Toto
500
Determine the area of the region bounded by
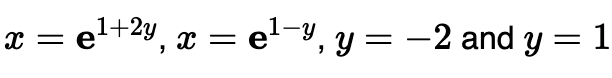
22.9983
500
Find the volume of the solid formed by revolving the region bounded by y=10-6x+x^2, y=-10+6x-x^2, x=1, x=5 about y = 8
938.289
500
Find the volume of region V when the region bounded by y=sin(πx/2), y=x^2
is revolved about x = 2
V=2.836
500
Let R be the region in the first quadrant bounded by the graph of y=x2, the line x=4, and the x-axis. R is the base of a solid whose cross sections perpendicular to the x-axis are equilateral triangles. What is the volume of the solid?
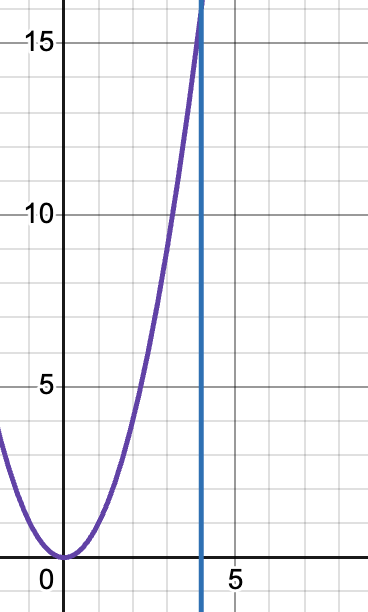
V=88.681
500
What is Mrs. Price's favorite anime?
Attack on Titan