Statistics
Linear Relationships
Geometry
Pythagoras Theorem
Lucky Dip
100
For the Box-Plot shown, state the range and IQR
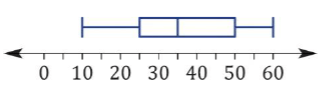
Range = 60 - 10 = 50
IQR = Q3 - Q1 = 50 - 25 = 25
100
Calculate the gradient of the line that connects (1, 8) and (3, 12)
m = (12-8)/(3-1) = 4/2 = 2
100
The triangle A(1,2), B(3,4), C(0,2) is reflected in the x-axis. State the coordinates of the image points A', B' and C'.
A' = (1,-2)
B' = (3,-4)
C' = (0,-2)
100
Determine if the following is a right-angled triangle:
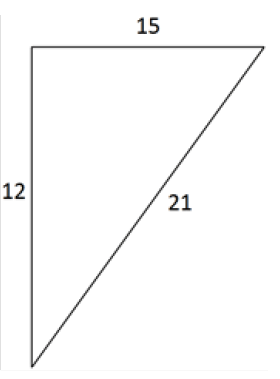
If right angled, then: c2 = a2 + b2
21^2 = 12^2 + 15^2
441 = 144 + 225
441 != 369
LHS != RHS
NOT a right-angled triangle
100
What date is your Semester test for Maths?
Thursday 24th November 2022
200
The data below represents the number of days students in class were absent from school this year. Represent it as a stem-and-leaf plot.
4 12 5 9 0 21 10 48 12
200
Find the equation of the line that is parallel to y = 2x +1 and passes through the point (1, 7).
Parallel means same gradient, so y = 2x + c.
Let x = 1, y = 7: 7 = 2x1 + c, so c = 5
So equation is y = 2x + 5
200
Given that the following triangles are similar, find the values of the pronumeral to 2 decimal places
Scale Factor = Image / Original = 2/11
therefore x = 12 xx 2/11 = 24/11 = 2.18cm
200
The following word related to Pythagoras' Theorem is misspelled. State the correct spelling:
High-pott-and-yous
Hypotenuse
200
Name this song & artist:
Never Gonna Give You Up - Rick Astley
300
Consider the following values:
3, 33, 15, 8, 33, 47, 22
State the mean, median and mode
Mean = 23
Median = 22
Mode = 33
300
Find the coordinates of the x and y intercepts for the line with the rule 3x + 2y = 12
For x-int, let y = 0
3x + 0 = 12
x = 4
x-int is (4,0)
For y-int, let x = 0
0 + 2y = 12
y = 6
y-int is (0,6)
300
Heron's formula for the area, A, of a triangle with side lengths a, b and c is given by:
A=sqrt(s(s-a)(s-b)(s-c)) and s=(a+b+c)/2
Find A when a=3cm, b=4cm and c=5cm
s=(a+b+c)/2 = (3 + 4 + 5)/2 = 6
A=sqrt(s(s-a)(s-b)(s-c))
A = sqrt(6(6-3)(6-4)(6-5))
A = sqrt(6xx3xx2xx1) = sqrt(36) = 6cm^2
300
The following represent the 3 side lengths of 3 triangles. For each triangle, determine whether it is a right-angle triangle or not.
A: 8cm, 15cm, 17cm
B: 9m, 16m, 25m
C: 12cm, 13cm, 50mm
A: YES - Satisfies Pythagoras Theorem
B: NO - Does not satisfy Pythagoras Theorem
C: YES - Satisfies Pythagoras Theorem (after making units the same)
300
Combine the following numbers together using addition, subtraction, multiplication and division to reach the target number. Each number can be used only once. You do not need to use all numbers.
100, 9, 6, 2, 8, 5 TARGET: 538
Many solutions exist. Some possible combinations:
5x100 + 2x(9+6) + 8 = 500 + 30 + 8 = 538
5x(100+6) + 8 = 530 + 8 = 538
5x(100+8) - 2 = 540 - 2 = 538
etc.
400
Write down a set of 6 integers with the mean < median < mode, and range = 10
Many solutions exist. One example:
1, 6, 8, 10, 11, 11.
(Mean = 7.8, Median = 9, Mode = 11, Range = 10)
400
Find the equation of the following line:
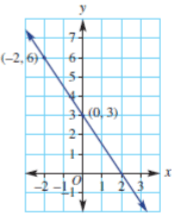
y = (-2)/3 x+3
400
Define the following triangles:
Equilateral, Isosceles, Scalene
BONUS: Which triangles can be right-angled?
Equilateral = All sides and angles are equal.
Isosceles = 2 sides are equal. 2 angles are equal.
Scalene = All sides and angles are different.
Right-Angled = A triangle where one of the internal angles is 90O. Therefore equilateral cannot be R-A (all angles 90O), isosceles can if the other two angles are 45O and scalene can if the other two angles are not 45O
400
Find the value of x, correct to 2 decimal places (if required):
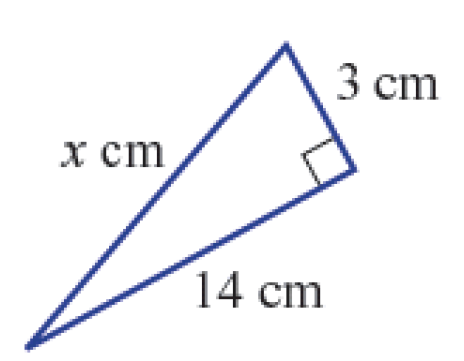
c^2 = a^2 + b^2
therefore x^2 = 14^2 + 3^2
therefore x^2 = 205
therefore x = sqrt(205)
x ~ 14.32cm
400
Recite pi to as many digits as you can. Most digits accurately remembered = 400 points, 2nd = 300 points, 3rd = 200 points, 4th = 100 points
pi =
3.1415 92653 58979 32384 62643 38327 95028 84197 16939 93751 05820 97494 45923 07816 40628 62089 98628 03482 53421 17067 9...
500
The following data represents the sale price of 10 houses in a Melbourne street. Calculate the upper and lower fences to determine if there are any outliers.
$510,000 $546,000 $560,000 $578,000 $595,000
$637,000 $693,000 $840,000 $952,000 $1,295,000
Min = 510,000, Q1 = 560,000, Med = 616,000 Q3 = 840,000, Max = 1,295,000, therefore IQR = Q3 - Q1 = 840,000 - 560,000 = $280,000
Lower Fence = Q1 - 1.5 x IQR = 560,000 - 1.5 x 280,000 = $140,000
Upper Fence = Q3 + 1.5 x IQR = 840,000 + 1.5 x 280,000 = $1,260,000
So $1,295,000 is an outlier because it is above the upper fence.
500
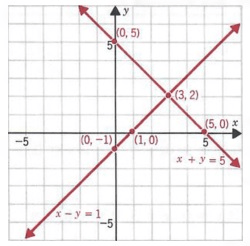
Sketch a graph of the following two lines on the same set of axes and find the coordinates of their intersection:
y = x - 1
y = -x + 5
500
A square starts with coordinates (-3, 1) , (2, 1) , (2, 6), (-3, 6). It then undergoes the following translations and transformations:
Translated 3 units up, reflected in the y-axis, translated 4 units left then rotated 180o clockwise about the origin. What are the new coordinates of the corners of the square?
(-3, 1) -> (-3, 4) -> (3, 4) -> (-1, 4) -> (1, -4)
(2, 1) -> (2, 4) -> (-2, 4) -> (-6, 4) -> (6, -4)
(2, 6) -> (2, 9) -> (-2, 9) -> (-6, 9) -> (6, -9)
(-3, 6) -> (-3, 9) -> (3, 9) -> (-1, 9) -> (1, -9)
500
Find the value of the pronumeral in the following composite shape, correct to 2 decimal places. Then, find its area (also correct to 2dp).
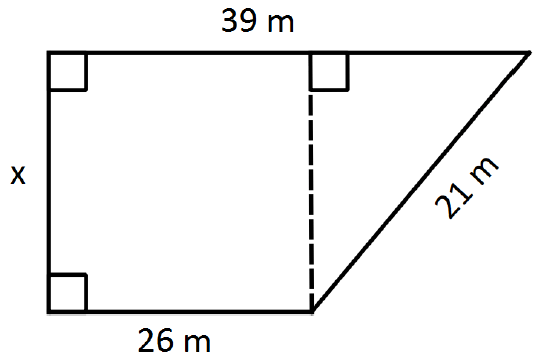
Area = Arectangle + Atriangle OR use Trapezium rule (A = (a+b)/2 x h)
A = (26+39)/2 xx sqrt(272) = 32.5 xx sqrt(272) = 536.00m^2 (allow 535.93m^2)
500
Name five year 8 maths teachers (100 points for each).
???