One-Step Inequalities
Two-Step Inequalities
Compound Inequalities
True/false statements
Absolute Value Evaluating, Equations, &Inequalities
100
Solve for x.
2x > 8
x > 4
100
True or False
When solving inequalities, flip the sign when multiplying both sides by a negative. Do not flip the sign when dividing both sides by a negative.
FALSE!!!!!
Flip the sign when multiplying or dividing both sides by a negative.
100
Solving Inequalities
4 < f + 6 < 5
What is:
-2 < f < -1
100
When solving absolute value equations and inequalities, you must first ISOLATE the absolute value. In other words, get the absolute value by itself.
True.
100
Solve:
|x - 4 | < 4
What is
0 < x < 8
x>0 AND x<8
200
Solve
X + 4 < -88
X < -92
200
Solving Inequalities
Solve for x.
2x - 5 > - 11
x > -3
200
Graph the inequality:
200
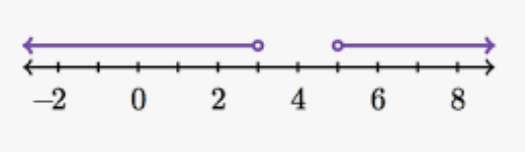
True or False?
-4 is included in the solution of
x > -20.
True.
-4 is greater than -20.
- 4 > -20
200
Solve:
3|c - 2| > 6
c < 0 OR C > 4
300
x/-5>2
x<-10
300
-4x+1>5
x<-1
300
Graph the inequality:
0<=x<3
300
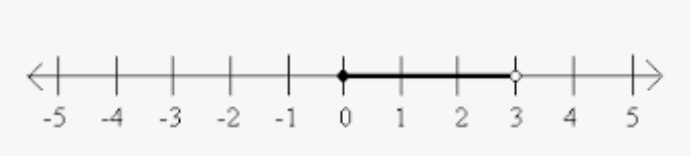
True or false:
If the variable is on the left side of the inequality and getting pointed to, shade right.
False.
If the variable is on the left side of the inequality and getting pointed to, shade left.
300
How would you rewrite the inequality to make the relationship make more sense for graphing?
-1>x> -5
-5<x< -1
400
z/30>70
Solve the Inequality
z > 2,100
400
Is 3 included in the solution to this inequality?
-9x + 1 > -71
Yes, because x must be less than 8.
400
Solve
-3p + 1 < -11 OR p + 4 < 6
p > 4 OR p < 2
400
True or False:
We use a closed circle to represent the inequalities < and >.
False
400
|2y - 9 | = 1
y = 5
y = 4
500
Solve the Inequality:
x-20 le -58
x le -38
500
Solve the inequality
11 < 2 - 3x
x < -3
or
-3 > x
500
-12 < -3x + 6 < -6
6 > x > 4
500
True or False: These two statements are the same
1<=x<4
1<=x and 4<x
False.
1<=x<4
500
Evaluate
|2x-4|<-2
No solution due to the fact that the absolute value cannot be less than a negative number.