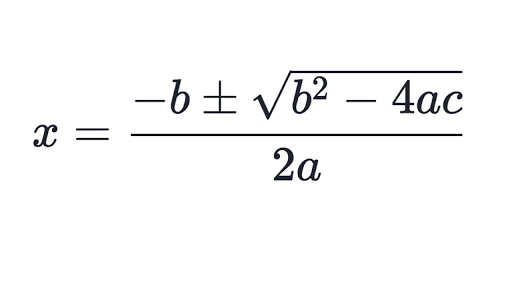Solving by factoring
Completing the square
Quadratic formula
Solving by taking square roots
Vertex & Standard form
Quadratic Features
100
What's the first step to solve quadratics by factoring
Step 1. Write the equation in standard form.
100
What's the first thing you have to do to complete the square?
Make sure that it is written in standard form and = to 0
ax2 + bx + c =0
(Remember, when y is 0 you will find the x-intercepts) (x,0)
100
When solving using the quadratic formula, what is the first 2 things you should do?
1st - make sure it is in standard form
(ax2 + bx + c = 0
2nd - Write down the values of;
a =
b =
c =
100
What do you have to do first to solve quadratics equation by taking square roots?
Isolate the square.
100
How is standard form written for a quadratic equation?
y = ax2 + bx + c
or
ax2 + bx + c = 0
100
Where is the vertex located?
At the point where the axis of symmetry cross the the parabola.
200
What do you have to do after you write the equation in the standard form?
Step 2. Factor completely.
Step 3. Use the zero product property.
Step 4. Solve each factor to get the x.
200
Rewrite in vertex form y = x2 − 6x by completing the square.
x2 − 6x = 0
x2 − 6x + 9 = 0 + 9
(x -3)2 = 9
y = (x-3)2 -9
200
Solve using the quadratic formula for this equation;
2m2 + 2m − 12 = 0
a = 2, b = 2, and c = -12
x = -(2) ±√ (2)2 - 4(2)(-12) / 2(2)
x = 2 and -3
200
Solve this equation by taking square roots.
x2 + 1 = 37
x2 = 36
x= 6, -6
200
How is vertex form written for a Quadratic Equation?
y = a(x-h)2 + k
or
a(x-h)2 + k = 0
200
What is the formula to find Axis of Symmetry?
x=-b/2a
300
Solve this quadratics by factoring to find the x-intercepts. (Hint: box method from unit 7 notes)
x2 + 2x = 3
x2 + 2x - 3 = 0
(x-1)(x+3) = 0
x = 1 or x = -3
300
Rewrite in vertex form y = x2 − 25 by completing the square.
x2 − 25 = 0
x2 = 25
(x)2 = 25
y = (x)2 - 25
300
Solve x2 + 4x - 21 = 0 by using quadratic formula.
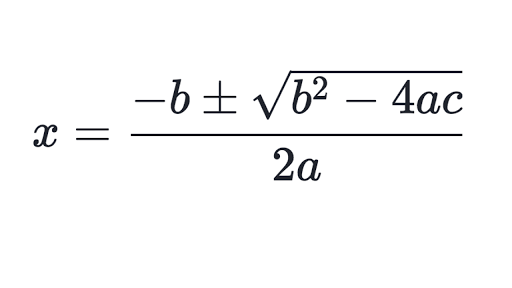
a = 1, b = 4, and c = -21
x = 3 or -7
300
Solve this equation by taking square roots.
x2 - 10 = 54
x2 = 64
x = 8 , -8
300
What is the formula for x-vertex coordinate when the quadratic equation is in standard form?
x =- b/(2a)
300
What is equation of the parent function of a quadratic equation?
y=x2
400
Solve this quadratics by factoring to find the x-intercepts. (Hint: box method from unit 7 notes)
X2 + 16 = 10x
x2 - 10x + 16 =0
(x-2)(x-8)
x = 2 or x = 8
400
Rewrite in vertex form x2 − 6x − 3 = 0 by completing the square.
x2−6x=3
x2−6x+(−3)2=3+9
(x−3)2 = 12
y =(x−3)2 - 12
400
Solve x2 - 8x + 14 = 0 using the quadratic formula.
a = 1, b = -8, and c = 14
x = -(-8) ±√ (-8)2 - 4(1)(14) / 2(1)
= 8 ±√ 8 / 2
= 5.41 and 2.59
400
Solve this equation by taking square roots.
(x-4)2 - 16 = 0
(x-4)2 = 16
x- 4 =± 4
x = 0 or 8
400
What is the formula for the vertex when the quadratic equation is in vertex form?
(-h, k)
400
When the discriminate is greater than one, how many solutions will your quadratic equation have?
TWO!
500
Solve this quadratics by factoring to find the x-intercepts. (Hint: box method from unit 7 notes)
18x2 - 3x = 6
18x2 - 3x -6 = 0
= 3(6x2 - x - 2) = 0
= 3(3x -2)(2x +1)= 0
= 3x -2 = 0 2x + 1 = 0
x = 2/3 or x -1/2
500
Rewrite in Vertex form x2 - 6x + 7 = 0 by using completing the square
x2 - 6x = -7
x2 -6x + (3)2 = -7 + 9
x2 -6x + 9 = 2
(x-3)2 = 2
y = (x-3)2 - 2
500
Solve 2x2 = 7x + 6 by using quadratic formula.
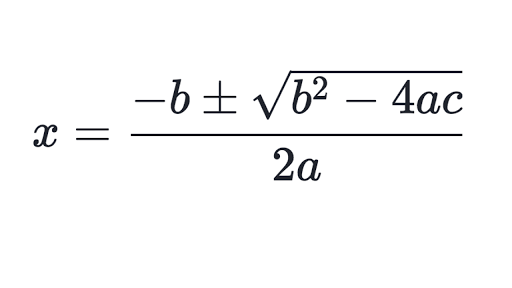
a = 2, b = -7, and c = -6
x = -(-7) ±√(-7)2 -4(2)(-6) / 2(2)
x = (7 ±√ 97) / 4
x =4.21 and -.71
500
Solve this equation by taking square roots.
(x+7)2 - 11 = 0
(x+7)2 = 11
x + 7 = ±√11
x = -3.68 and -10.32
500
What does "a value" represent on both vertex and standard form?
("a value"uarr or darr)/(1harr)
500
When the discriminate is less than zero, how many times does the parabola cross the x-axis?
None.